Hello, my dear friends. I am back with my Visually Explained! article series. Today we will look into some of the very fundamental concepts from Vector Calculus. So as I have told before, the focus of Visually Explained! series is on physical intuition rather than on explaining the equations and detailed math. So let us begin.
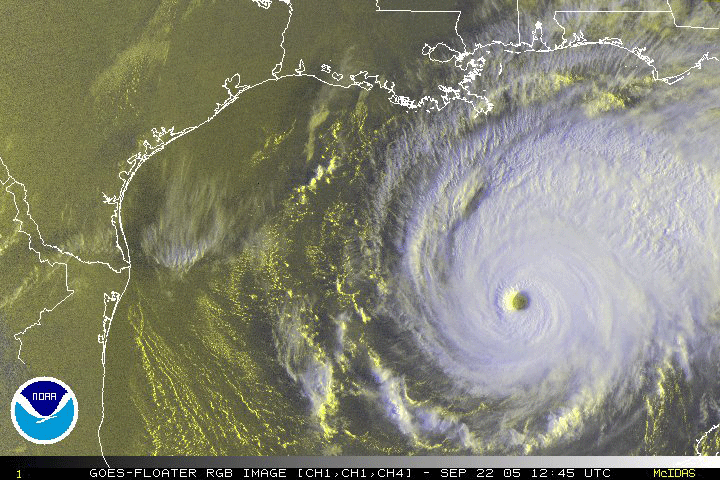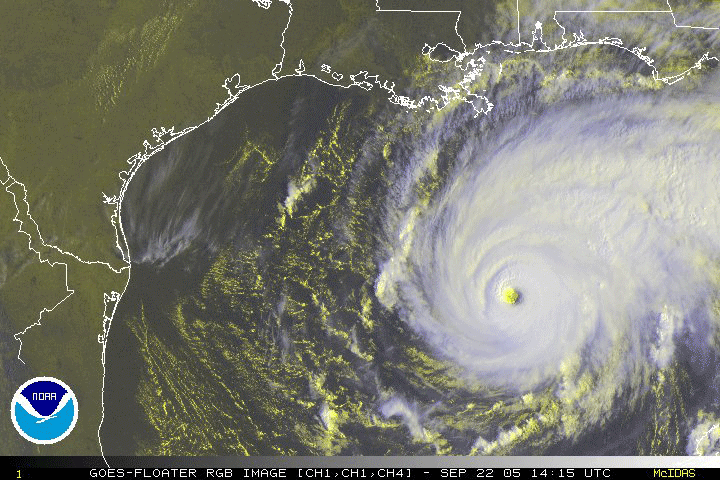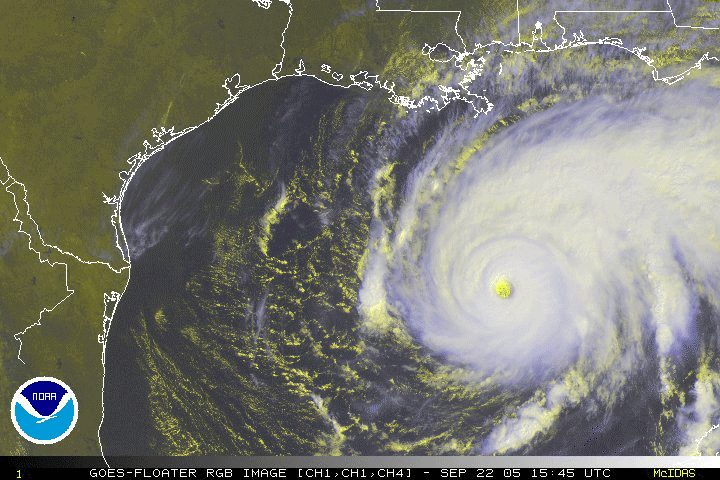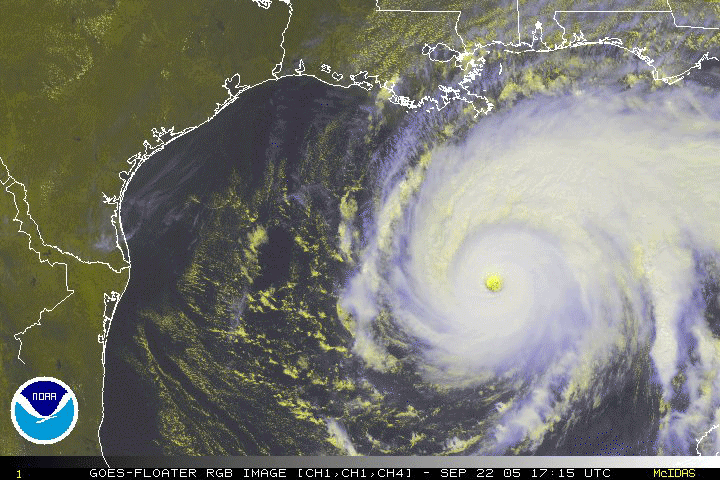
Source: Wikimedia, By NOAA [Public domain], via Wikimedia Commons
The above gif animation shows a cyclone named Rita, which is a real life example of Vector fields. We will come to this later.
PLAN FOR TODAY
I will give you a basic idea about the following concepts:
- Introduction on scalar and vector quantities
- Dot product and Cross product
- Gradient
- Divergence
- Curl
I will mostly motivate concepts by giving examples rather than definitions. There will be equations of course, but it is not necessary to follow this article.
Introduction
SCALAR: A scalar is some quantity which has only a magnitude associated with it. For example: Take the case of temperature. It can be 32o Celsius. One value is only associated with a scalar. Now let us see what is a scalar field. Below is an image of annual mean temperature in the world with respect to different regions. This is a scalar field:
Image Source: Wikimedia Commons, License: CC BY-SA 3.0
VECTOR: A vector is a variable which has magnitude and a direction associated with it. For example below is a vector 
, where 
is the unit vector(vector with length 1) in the X direction and 
is the unit vector in the Y axis:
Image Source: Wikimedia Commons, License: CC BY-SA 3.0
In the above image the magnitude of the vector is its length and direction is obvious. So when it comes to vector field, you may think it as a flow of vectors like below(electric field from positive to negative charge. This is a static scenario, when field doesnot change with time.):
Image Source: Wikimedia Commons, License: CC BY-SA 3.0
Now let us think about time varying fields. So the first image I showed about the cyclone was one example. A second one is shown here(these are electric fields from a dipole-antenna):
Image Source: Wikimedia Commons, License: CC0 1.0 Universal (CC0 1.0) Public domain dedication
Dot product and Cross product
Now let us very fundamental concepts called dot-product and cross-product which are necessary for the understanding of gradient, divergence and curl etc. Dot product is a vector product which tells how similar two vectors are.
Dot product of 2 vectors A and B are defined as 
which means dotproduct of vectors A and B is equal to magnitudes of A times B times coine of angle between A and B. If that angle is 0, the dot product is the product of A times B. If the angle is 180 degrees (A and B looking opposite directions), then the dot product is -A times B. If they are perpendicular the dot product is zero. It is a measure of similarity in direction of vectors.
Cross product is another kind of vector product. Dot product(aka scalar prosuct) of 2 vectors gave us a scalar! But cross product(aka vector product) gives you a vector. It is defined as 
The 
is a unit vector(vector with unit length) in the direction perpendicular to A a nd B vectors. To get the direction of unit vector here you can use the right hand thumb rule as below:
Image Source: Wikimedia Commons, License: CC BY-SA 3.0
So the n-hat is the direction of A X B (A cross B), the thumb's direction in the above image.
GRADIENT
Imagine a scalar field. Suppose that you are on a surface (which is a scalar field of heights in the z-direction) like shown in figure (a) below:
Image Source: Wikimedia Commons, License: CC BY-SA 3.0
Gradient tells you to which direction you have to move towards the higher elevation. The magnitude corresponding to this direction is the slope at that particular point. See the figure above. If you are are on a crest or trough, there exists no slope (these are zero vectors). But as you move away from trough there is an outward flux of vectors towards higher elevations and inward flux of vectors in case of a crest(peak). The resulting output is a vector field. Mathematically you have to define a gradient operator for these purposes. It is done(for 2-dimensional cases) as below:
i and j are the unit vectors in x and y dimensions.
DIVERGENCE
Divergence actually operates on some vector field to give a scalar field output. Divergence measures if there is any source or sink exists. I will explain it using the following figure:
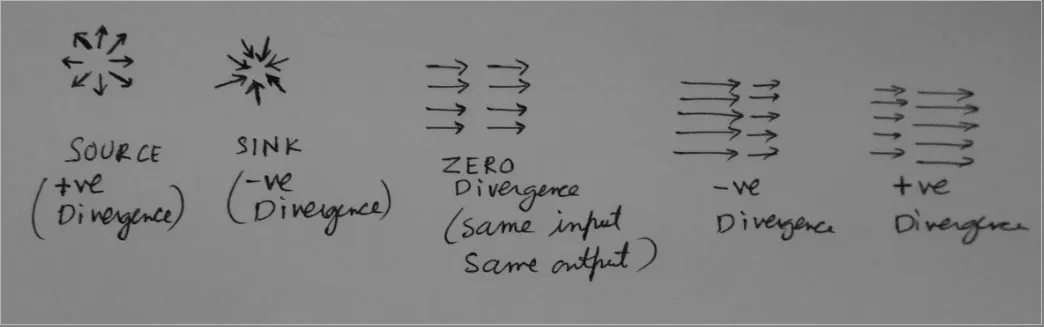
Mathematically, you define divergence as below:
It is like the dot-product of the del-operator and F vector field.
CURL
Curl measures if there are any "rotations" (like eddies) in the vector field. For example, a cyclone in the vector field has corresponding curls. The Curl of a vector field is another vector field. So the direction of the curl vectors is found according to the right-hand thumb rule. In the image below, I is the curl vector and B is the input vector field where you have a rotation.
Image Source: Wikimedia Commons, License: CC BY-SA 3.0
The Curl of a vector field is the cross product of the gradient operator with the vector field:
Intuitively you can check if a vector field exhibits a curl using an infinitesimal rotor. Let me denote this rotor as a star in the figure below. The question I ask is whether the rotor rotates. If so, in which direction?:
If the thumb points outside the paper, I call it positive curl. The Second case in the above figure, you have same vectors hitting rotor so that it gets locked.
So I think this is enough for today. Please do ask questions in replies, if you have any doubts. I will stop with a short funny music video (from youtube) illustrating Maxwell's laws where will see curl and divergence etc.
About #steemSTEM
#steemSTEM is an active community which focuses on promoting original, nicely written and informative articles on Science, Technology, Engineering and Mathematics in Steemit. steemSTEM is the best science community I know of in the whole of steemit. So do check out the articles which come with this hashtag #steemSTEM. And also don't forget to join the steemSTEM discord channel: https://discord.gg/BZXkmWw
@suesa 's recent mentorship programme for beginners:
I have something to share with beginners in steemSTEM. If you are a beginner steemian like me and wants to improve, @suesa gives a mentorship programme. So read the article below and apply for it :
@suesa/suesa-s-mentorship-round-2
If you like my content please upvote and resteem it. Below is my recent post, you may find interesting:
The history and mystery of Belousov-Zhabotinsky reaction: Did it violated 2nd law of thermodynamics